Expand (x y z)^2 formula 234438

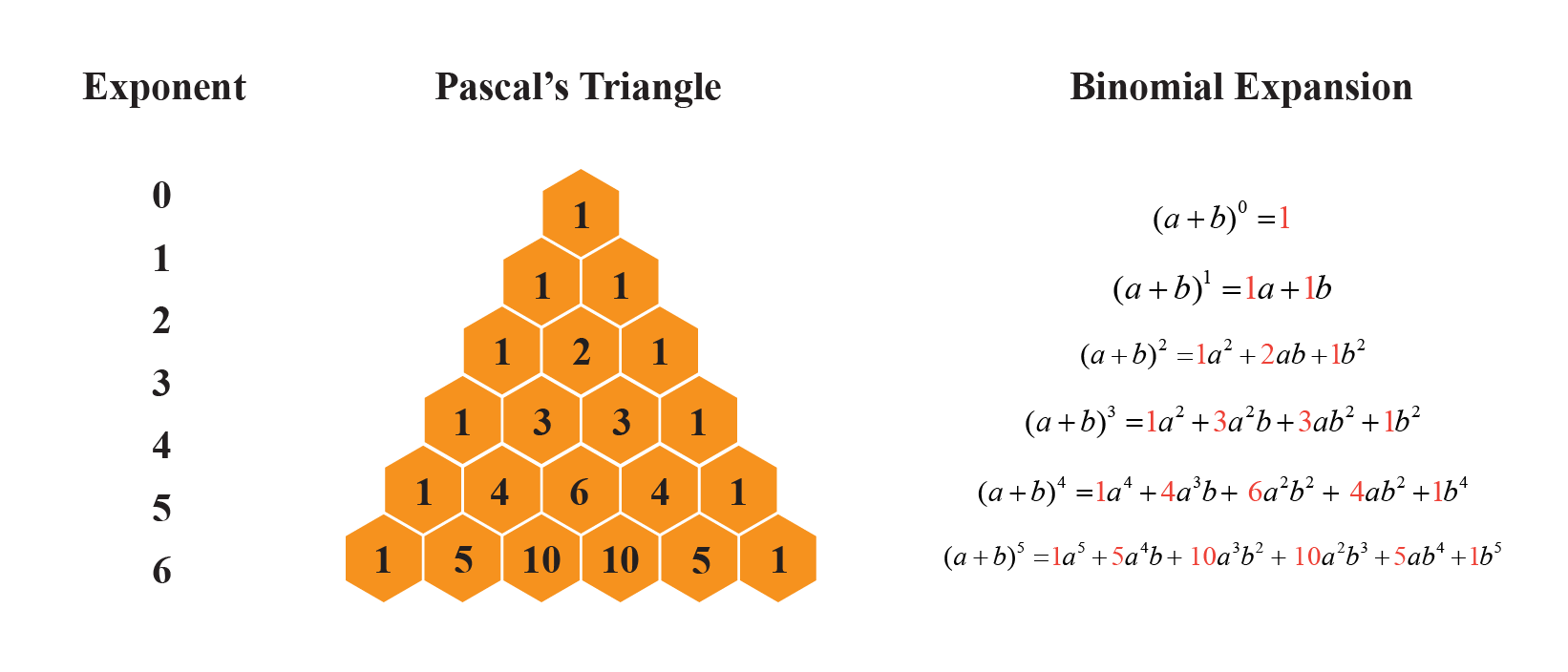
Mar 04, 16 · #(xy)^6=x^66x^5y15x^4y^2x^3y^315x^2y^46xy^5y^6# Explanation The Binomial Theorem gives a time efficient way to expandFind a power series expansion about x 0 for a general solution to the given differential equation Your answer should include a general formula for the coefficientsTaylor's Formula Consider the function f(x,y) Recall that we can approximate f(x,y)withalinearfunction 2 x @ @x y @ @y It turns out that you can easily get the coecients of the expansion from Pascal's Triangle 1 11 121 1331

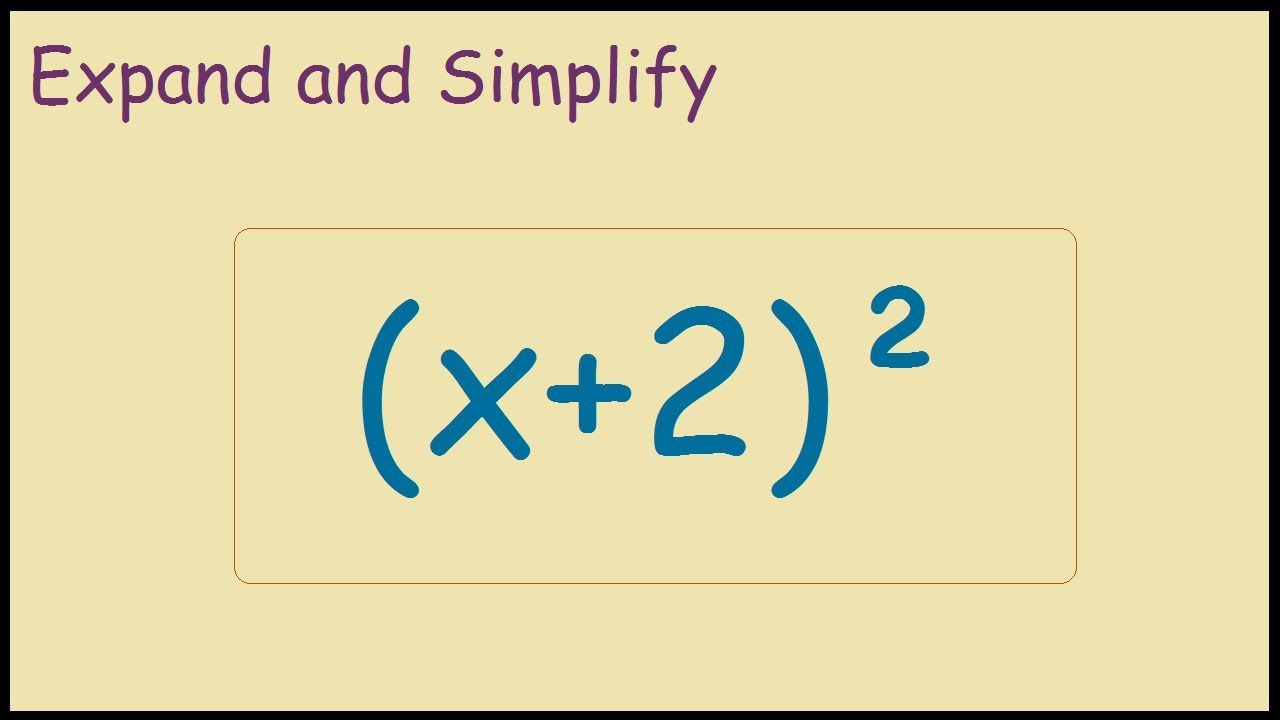
X 2 2 Expand And Simplify Using Foil Method Youtube

Expand (x y z)^2 formula

Expand (x y z)^2 formula-The calculator can also make logarithmic expansions of formula of the form `ln(a^b)` by giving the results in exact form thus to expand `ln(x^3)`, enter expand_log(`ln(x^3)`), after calculation, the result is returned The calculator makes it possible to obtain the logarithmic expansionX 3 , obtained by taking the Taylor series of (1 x) α centered at the origin If α is a nonnegative integer n, observe that the coefficient of x k is (n k) for k

Unit 2 Algebra Mathematics 9 1 Igcse Year Ppt Download

Each term r in the expansion of (x y) n is given by C(n, r 1)x n(r1) y r1 Example Write out the expansion of (x y) 7 (x y) 7 = x 7 7x 6 y 21x 5 y 2 35x 4 y 3 35x 3 y 4 21x 2 y 5 7xy 6 y 7 When the terms of the binomial have coefficient(s), be sure to apply the exponents to these coefficients Example Write out the$\begingroup$ Could you figure it out if you rewrote it as $((2x3y)4z)^n$ and then used your formula for $(xy)^n$ (perhaps many times)?Free expand & simplify calculator Expand and simplify equations stepbystep This website uses cookies to ensure you get the best experience By using this

That is, for each term in the expansion, the exponents of the x i must add up to n Also, as with the binomial theorem, quantities of the form x 0 that appear are taken to equal 1 (even when x equals zero) In the case m = 2, this statement reduces to that of the binomial theorem Example The third power of the trinomial a b c is given byOn the Binomial Theorem Problem 1 Use the formula for the binomial theorem to determine the fourth term in the expansion (y − 1) 7 Show Answer Now, we have the coefficients of the first five terms By the binomialExpand the trigonometric expression cos(x y)Simplify the cos function input x y to x or y by applying standard identities

Remainder of x^32x^25x7 divided by x3;Sep 08, 17 · mysticd Answer (xyz)² = x²y²z²2xy2yz2zx Stepbystep explanation (xyz)² = x(y)(z)² = x²(y)²(z)²2x(y)2(y)(z)2(z)x /* By alge braic identity (abc)²=a²b²c²2ab2bc2ca */X²yy²xy²zxyzx²zz²xz²yxyz (Just spilliting the terms for factorization) take y common from first 4 terms and take z common from last 4 terms x²yy²xy²zxyzx²zz²x²yxyz= y (x²xyyzzx)z (y (x²xyyzzx)z (x²zxyzxy)) Now in this expression "x²xyyzzx" take x common in first two terms and z common in last two terms And in this "x²zxyzxy" take x

Module 2 Implicit Differentiation Equations Function Mathematics

Solved Problem 3 10 Points Consider The Ode Y Xy Chegg Com

For any fixed α ∈ C, we have the Newtonian expansion (1 x) α = 1 α x α (α − 1) 2!Given x^2y^2z^2=xyyzzx (1)multiplying by (xyz) on both sidesx^2y^2z^2 (xyz)=(xyyzzx ) (xyz)expand the above equationsx^3y^3z^3xy^2x^2 yyz^2y^2 zxz^2x^2 z=x^2 yxyzzx^2xy^2 y^2 zxyzxyzSimplify (xy)^2 Rewrite as Expand using the FOIL Method Tap for more steps Apply the distributive property Apply the distributive property Apply the distributive property Simplify and combine like terms Tap for more steps Simplify each term

The Binomial Theorem Explained With A Special Splash Of Pascal S By Brett Berry Math Hacks Medium

If X 2 Y 2 14 And Xy 5 Find The Value Of 1 2x 1 2y 2 Brainly In

View more examples » Access instant learning tools Get immediate feedback and guidance with stepbystep solutions and WolframApr 09, 18 · Expand the first two brackets (x −y)(x − y) = x2 −xy −xy y2 ⇒ x2 y2 − 2xy Multiply the result by the last two brackets (x2 y2 −2xy)(x − y) = x3 − x2y xy2 − y3 −2x2y 2xy2 ⇒ x3 −y3 − 3x2y 3xy2 Always expand each term in the bracket by all the other terms in the other brackets, but never multiply two or more terms in the same bracket1) where P ℓ is the Legendre polynomial of degree ℓ This expression is valid for both real and complex harmonics The result can be proven analytically, using the properties of the Poisson kernel in the unit ball, or geometrically by applying a rotation to the vector y so that it points along the z axis, and then directly calculating the righthand side In particular, when x = y , this

General And Middle Term Binomial Theorem Formula Videos Examples

X 2 2 Expand And Simplify Using Foil Method Youtube

Expand the following product (3 x 1) (2 x 4) `(3x1)(2x4)` returns `3*x*2*x3*x*42*x4` Expand this algebraic expression `(x2)^3` returns `2^33*x*2^23*2*x^2x^3` Note that the result is not returned as the simplest expression in order to be able to follow the steps of calculations To simplify the results, simply use the reduce functionSolutionShow Solution ( x y z ) 2 = x 2 y 2 z 2 2 (x) (y) 2 (y) (z) 2 (z) (x) = x 2 y 2 z 2 2xy 2yz 2zxQuotient of x^38x^217x6 with x3;

Solved 3 4 6 Jake Buys A Bag Of Popcorn At A Movie Theater He Eats Half Of The Popcorn During The 15 Minutes Of If X 15 What Is The Value Course Hero

Cube Of A Binomial Calculator Solved Examples Cuemath

In the previous section you learned that the product A(2x y) expands to A(2x) A(y) Now consider the product (3x z)(2x y) Since (3x z) is in parentheses, we can treat it as a single factor and expand (3x z)(2x y) in the same manner as A(2x y) This gives us If we now expand each of these terms, we haveNumber of terms the expansion of (x y z w) 10 The problem given to understand these types of questions is an expansion of a quadrinomial or a polynomial that has four terms Going back to a property of the binomial theorem, the sum of the powers of its variables on any term is equal to n, where n is the exponent on (x y)How to expand the square of a trinomial?

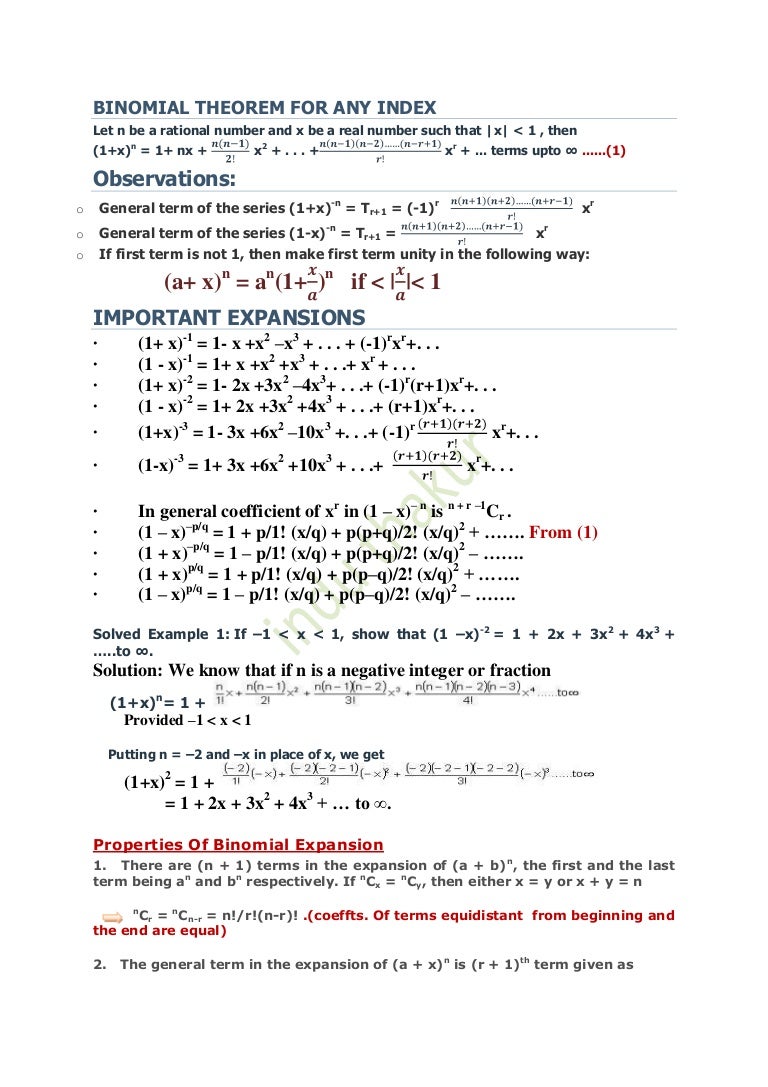
Binomial Theorem For Any Index

Binomial Theorem Solutions Examples Videos

Note that we have to keep the sum of powers in each of the combinations of $x,y,z$ to $n$, so it will be reduced Now replace $a$ and $b$ by $x$ and $(yz)$ respectively So total number of terms should be $123\cdots(n1)=\dfrac{(n1)(n2)}{2}$(x y z) 6?A complex number z = x yi will lie on the unit circle when x 2 y 2 = 1 Some examples, besides 1, –1, i, and –1 are ±√2/2 ± i√2/2, where the pluses and minuses can be taken in any order They are the four points at the intersections of the diagonal lines y = x and y = x with the unit circle

Unit 2 Algebra Mathematics 9 1 Igcse Year Ppt Download

Write The Following In The Expanded Form 3x Y Z 2 Ii

Feb 25, 16 · =x^55x^4z10x^3z^210x^2z^35xz^4z^5 The binomial theorem states that (xy)^nsum_(r=1)^n ""^nC_rx^(nr)y^r therefore (xz)^5=sum_(r=1)^5""^5C_rx^(5r)z^r =""^5C_0x^5""^5C_1x^(51)z^1""^5C_2x^(52)z^2""^5C_3x^(53)z^3""^5C_4x^(54)z^4""^5C_5x^(55)z^5 =x^55x^4z10x^3z^210x^2z^35xz^4z^5Dec 16, 15 · =1 (1/2)x (3/8)x^2 (5/16) x^3 In the binomial expansion formula for (1x)^n = 1 nx (n(n1))/(2!)x^2 substitute x for x and 1/2 for n The resultWhat is the coefficient of the x 2 y 2 z 2 x^2y^2z^2 x 2 y 2 z 2 term in the polynomial expansion of (x y z) 6?

The Greatest Binomial Coefficient In The Expansion Of X 3 2 Y

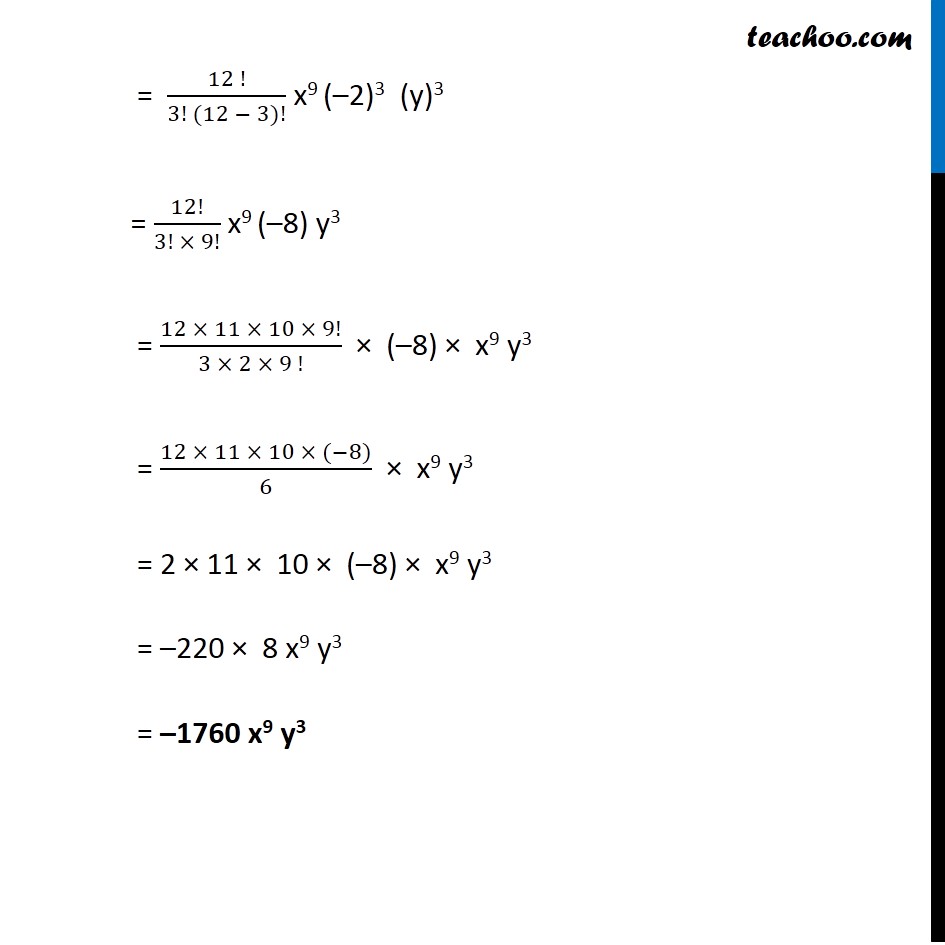
Ex 8 2 5 Find 4th Term Of X 2y 12 Chapter 8 Class 11

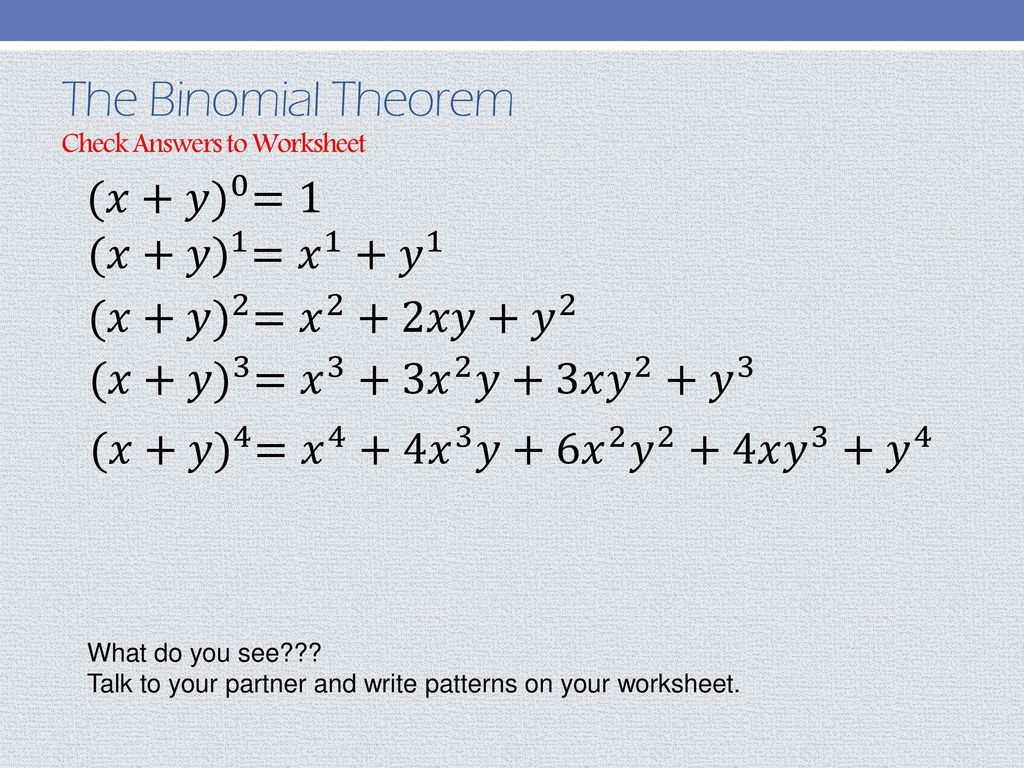
For $z = x iy$, we have, for example $\Re (z)=x$ and $\Im (z)=y$ $\Re (z^2)= x^2y^2$ and $\Im (z^2)=2xy$ $\Re(z^3)=x^33xy^2$ and $\Im (z^3)=3yx^2y^3$ I know that IUNIT 149 PARTIAL DIFFERENTIATION 9 TAYLOR'S SERIES FOR FUNCTIONS OF SEVERAL VARIABLES 1491 THE THEORY AND FORMULA Initially, we shall consider a function, f(x,y), of two independent variables, x, y, and obtain(x y) 2 = x 2 2xy y 2 (x y) 3 = x 3 3x 2 y 3xy 2 y 3 (x y) 4 = x 4 4x 3 y 6x 2 y 2 4xy 3 y 4;

Unit 2 Algebra Mathematics 9 1 Igcse Year Ppt Download

Zytenze Xy Male Expansion Formula Testosterone Support Secret Men Blood Flow Expansion Use Zytenz E To Help Boost Male Energy Recovery Drive Libido Circulation Nutrient Delivery Health Personal Care Amazon Com

Proof Let x y = k then, (x y z)2 = (k z)2 = k2 2kz z2 (Using identity I) = (x y)2 2 ( x y)z z2 = x2 2xy y2 2 xz 2yz z2 = x2 y2 z2 2xy 2yz 2zx i hope it is aExpand (xy)^2 Rewrite as Expand using the FOIL Method Tap for more steps Apply the distributive property Apply the distributive property Apply the distributive property Simplify and combine like terms Tap for more steps Simplify each term Tap for more steps Multiply by Multiply by Add and149 Taylor's Formula for Two Variables 2 Define F(t) = f(ath,btk) The Chain Rule gives F0(t) = f x dx dt fy dy dt = hfx kfy Since fx and fy are differentiable (by assumption), F0 is a differentiable function of t and F00 = ∂F0 ∂x

Prove That Sinx Siny Cosx Cosy Cot X Y 2 Brainly In

Chapter 3 More About Factorization Ppt Download

An outline of Isaac Newton's original discovery of the generalized binomial theorem Many thanks to Rob Thomasson, Skip Franklin, and Jay Gittings for theirZ = x iy, since z becomes the combination rcosµ irsinµ, which suggests that the combination may be interesting to look at (unit circle has r = 1) This turns out to be a very important unification and simplification of many results in both trigonometry and calculus, in which the formula leads us to correct manipulationsAlgebra Simplify (xyz)^2 (x y z)2 ( x y z) 2 Rewrite (xy z)2 ( x y z) 2 as (xyz)(xyz) ( x y z) ( x y z) (xy z)(xyz) ( x y z) ( x y z) Expand (xyz)(xyz) ( x y z) ( x y z) by multiplying each term in the first expression by each term in the second expression

Solved 2 4 Points Use The Binomial Theorem A To Expa Chegg Com

Applied Differential Equations 2280 Sample Final Exam Tuesday 2 May 06 4 30 8 00pm

Zz = x2 y2;(x y z) 2 = x 2 y 2 z 2 2xy 2yz 2zx (x 2y 4z) 2 = x 2 (2y) 2 (4z) 2 2(x)(2y) 2(2y)(4z) 2(4z)(x) = x 2 4y 2 16z 2 4xy 16yz 8xz(4) This modulus is equivalent to the euclidean norm of the 2D vector (x;y), hence it obviously satisfy the triangle inequality jz 1 z 2j jz 1j jz 2j However we can verify that jz 1z 2j= jz 1jjz 2j Division z 1 z 2 = z 1z 2 z 2z 2 = (x 1 iy 1)(x 2 iy 2) x2 2 y2 2 = x 1x 2 y 1y 2 x2 2 y2 2 i x 2y 1 x 1y 2 x2 2 y2

Write The Following In The Expanded Form A B B C C A 2 I

How Can We Expand X Y 1 2 Youtube

Although the formula above is only applicable for binomials raised to an integer power, a similar strategy can be applied to find the coefficients of any linear polynomial raised to an integer powerExpanding (x y) n yields the sum of the 2 n products of the form e 1 e 2 e n where each e i is x or y Rearranging factors shows that each product equals x n−k y k for some k between 0 and n For a given k, the following are proved equal in succession the number of copies of x n−k y k in the expansionExpand (xyz)^2 (x − y − z)2 ( x y z) 2 Rewrite (x−y −z)2 ( x y z) 2 as (x−y−z)(x−y−z) ( x y z) ( x y z) (x−y− z)(x−y−z) ( x y z) ( x y z) Expand (x−y−z)(x−y−z) ( x y z) ( x y z) by multiplying each term in the first expression by each term in the second expression

Expand And Simplify Binomial Squares 2x 3y 2 Youtube

Taylor Series Expand X 2y 3y 2 In The Powers Of X 1 And Y 2 Up To 3 Degree Terms Youtube

Algebra Expand (xyz)^2 (x − y z)2 ( x y z) 2 Rewrite (x−y z)2 ( x y z) 2 as (x−yz)(x−yz) ( x y z) ( x y z) (x−y z)(x−yz) ( x y z) ( x y z) Expand (x−yz)(x−yz) ( x y z) ( x y z) by multiplying each term in the first expression by each term in the second expressionIntroduction to x plus y whole cube identity with example problems and proofs to learn how to derive xy whole cube formula in mathematicsSep 23, 16 · To expand #(xy)^6#, use the coefficients in front of # x^6y^0#, #color(white)(aa)x^5y^1#, #color(white)(aa)x^4y^2#, etc, with the exponent of #x# starting at 6 and decreasing by one in each term, and the exponent of #y# starting at 0 and increasing by one in each term Note the sum of the exponents in each term is 6

11 Partial Differential Equations Partial Differential Equation Differential Calculus

Find The Middle Term In The Expansion 2 By 3 X Minus 3 By 2 Y Whole Power Brainly In

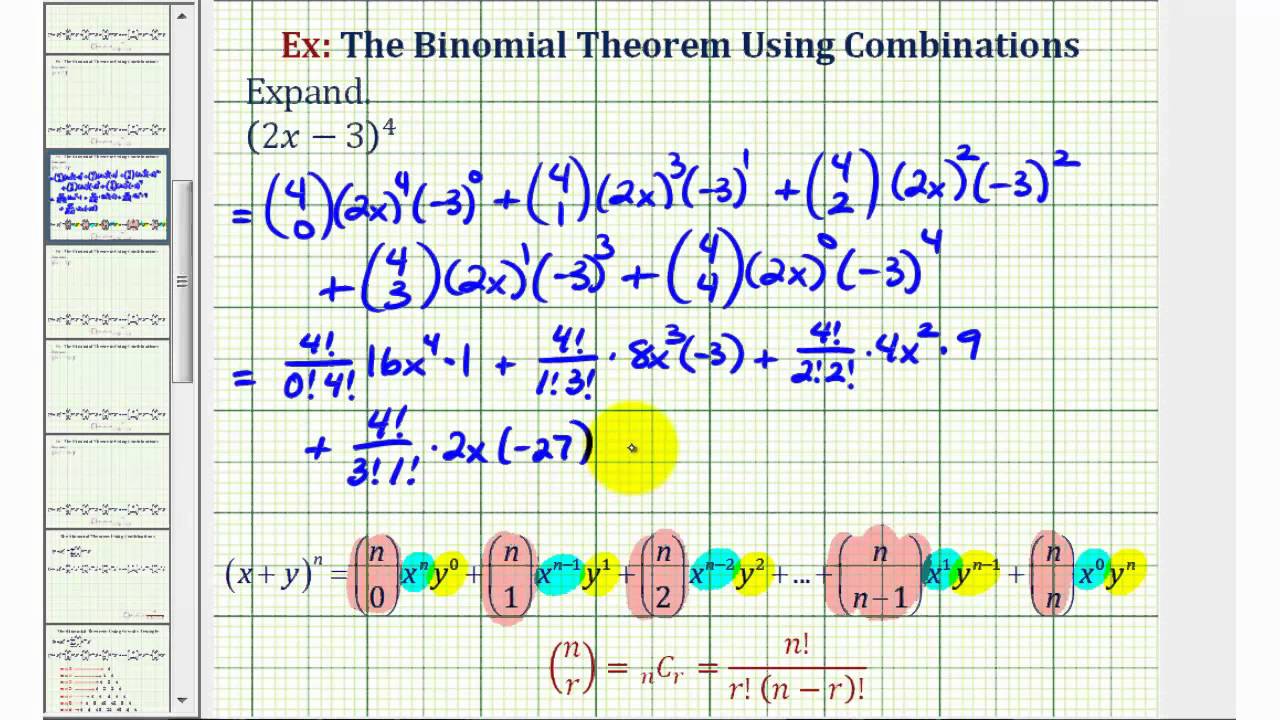
When we expand latex{\left(xy\right)}^{n}/latex by multiplying, the result is called a binomial expansion, and it includes binomial coefficients If we wanted to expand latex{\left(xy\right)}^{52}/latex, we might multiply latex\left(xy\right)/latex by itself fiftytwo times This could take hours!Free PreAlgebra, Algebra, Trigonometry, Calculus, Geometry, Statistics and Chemistry calculators stepbystepIn mathematics, the binomial coefficients are the positive integers that occur as coefficients in the binomial theoremCommonly, a binomial coefficient is indexed by a pair of integers n ≥ k ≥ 0 and is written () It is the coefficient of the x k term in the polynomial expansion of the binomial power (1 x) n, and is given by the formula =!!()!For example, the fourth power of 1 x is

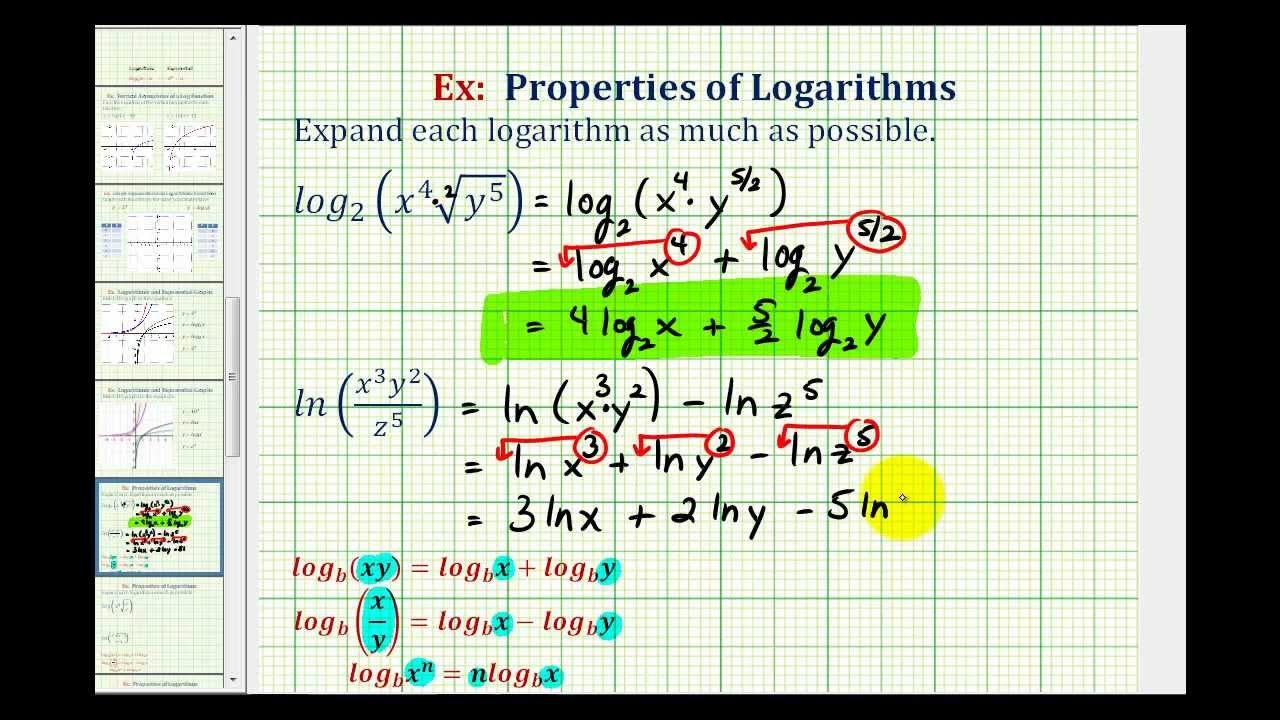
Logarithms Product Rule Solutions Examples Videos Worksheets Games Activities

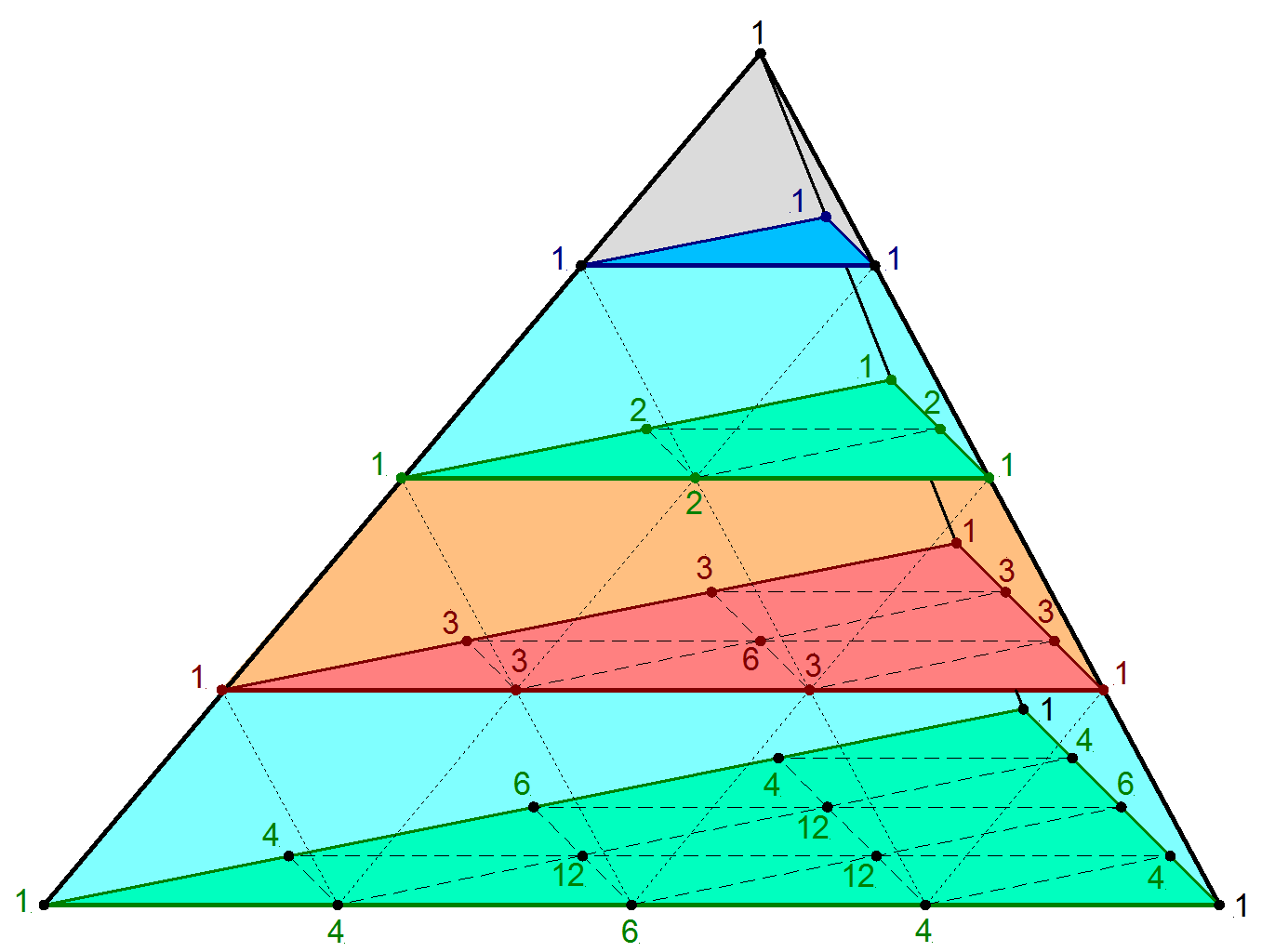
Pascal S Pyramid Wikipedia

Please prove that $\cos (x iy) = \cos x \cosh y i\sin x \sinh y$ and $\cos (x iy) = \cos x \cosh y i\sin x \sinh y$ I admit that iI don't even know how to start proving this Honestly, I don't know much about complex numbers and it's hard for me to understand the answers on the net$\endgroup$ – Michael Burr Sep 15 '17 at 924 $\begingroup$ I have thought of this by substituting 3y4z=z, we know the formula of (xy)^n , it i want some gemeral ways $\endgroup$ – Samar Imam Zaidi SepExpand polynomial (x3)(x^35x2) GCD of x^42x^39x^246x16 with x^48x^325x^246x16;

Ex 2 5 6 Write The Following Cubes In Expanded Form Ex 2 5

Rd Sharma Solutions For Class 8 Chapter 6 Algebraic Expressions And Identities Download Free Pdf

X 2 α (α − 1) (α − 2) 3!The square of the sum of three or more terms can be determined by the formula of the determination of the square of sum of two terms (z) 2 2 (3x) (2y) 2 (2y) (z) 2 (z) (3x) = 9x 2 4y 2 z 2 – 12xy 4yz – 6zx 2 Simplify a b c = 25 and ab bc ca = 59 Therefore, a 2 b(x y z) 2 = x 2 y 2 z 2 2xy 2xz 2yz (x y z) 2 = x 2 y 2 z 2 2xy 2xz 2yz

Factoring Two Variable Quadratics Rearranging Video Khan Academy

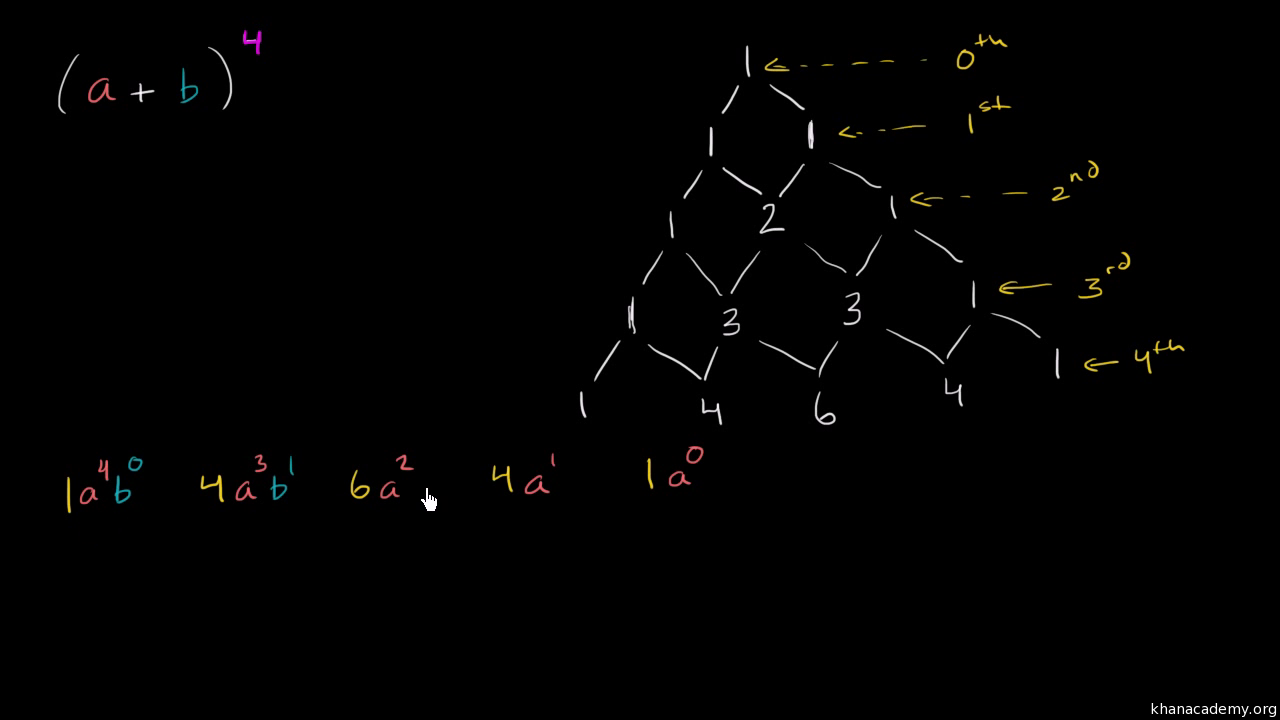
Pascal S Triangle And Binomial Expansion Video Khan Academy

So, the expansion of (x 2y z) 2 is x 2 4y 2 z 2 4xy 4yz 2xz a minus b plus c Whole Square Formula To get formula / expansion for (a b c) 2, let us consider the formula / expansion for

Verify That X3 Y3 Z3 3xyz 1 2 X Y Z X Y 2 Y Z 2 Z X 2 Ieep2 Polynomial Mathematics Of Computing Learn All Concepts Of Polynomials Class 9 With Videos Diana Images

The Binomial Theorem Fractional Powers Expanding 1 2x 1 3 Youtube

Rd Sharma Solutions For Class 8 Chapter 6 Algebraic Expressions And Identities Download Free Pdf

03 Solving Equations With Two Variables In Algebra Part 3 Algebra 1 Course Unit 7 Slope And The Equation Of A Line Math Tutor Public Gallery

Igcse Cambridge 0580 Quadratic Simultaneous Equations Complete Square Expand Triple Brackets Youtube

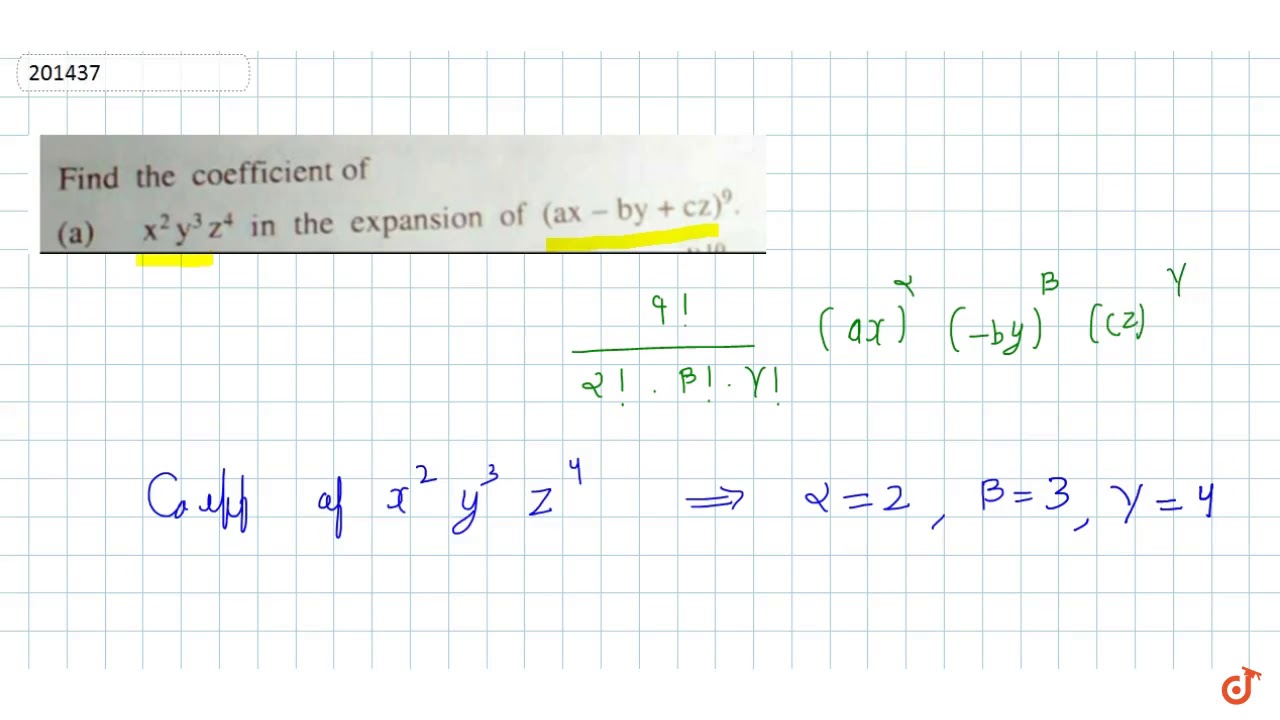
Find The Coefficient Of X 2 Y 3 Z 4 In The Expansion Of Ax By Cz 9 Youtube

Video Binomial Expansion Nagwa

Binomial Expansion Equation Genetics Page 1 Line 17qq Com

14 2 Limits And Continuity

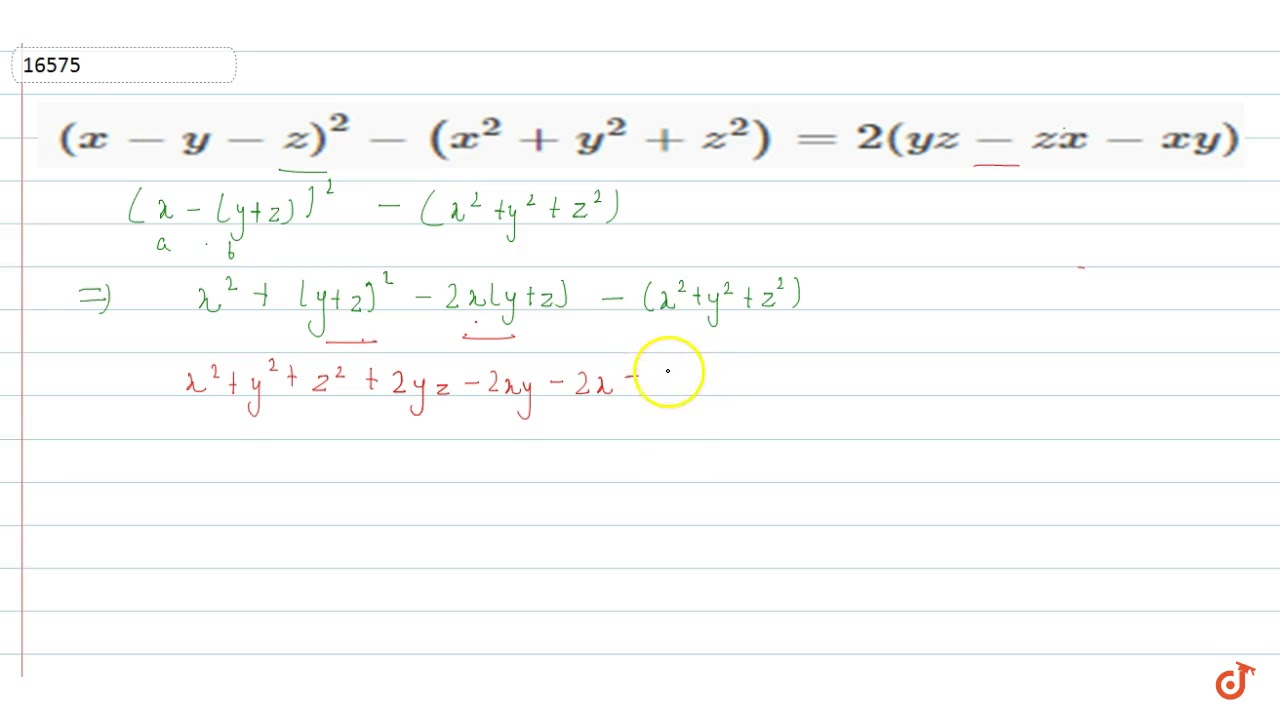
X Y Z 2 X 2 Y 2 Z 2 2 Yz Zx Xy Youtube

Wanted To Find Taylor Series For A Function Thanks Wolfram Alpha Math

Expand 2x Y 5 With The Help Of Binomial Theorem

3 6 Pascal S Triangle And The Binomial Theorem Mathematics Libretexts

Solution What Is The Sum Of The Coefficients In The Expansion X Y Z 8

Algebraic Identities Mathematics 1a Studocu

Conic Section From Expanded Equation Ellipse Video Khan Academy

Find Middle Term For Even Power Binimial Expansion Youtube

3 6 Pascal S Triangle And The Binomial Theorem Mathematics Libretexts

The Expanded Form Of X Y X Y Is A

Expand Using Identity 3 X Minus 2 Y Z Whole Square Brainly In

X Y Z Whole Square Formula A Pictures Of Hole 18

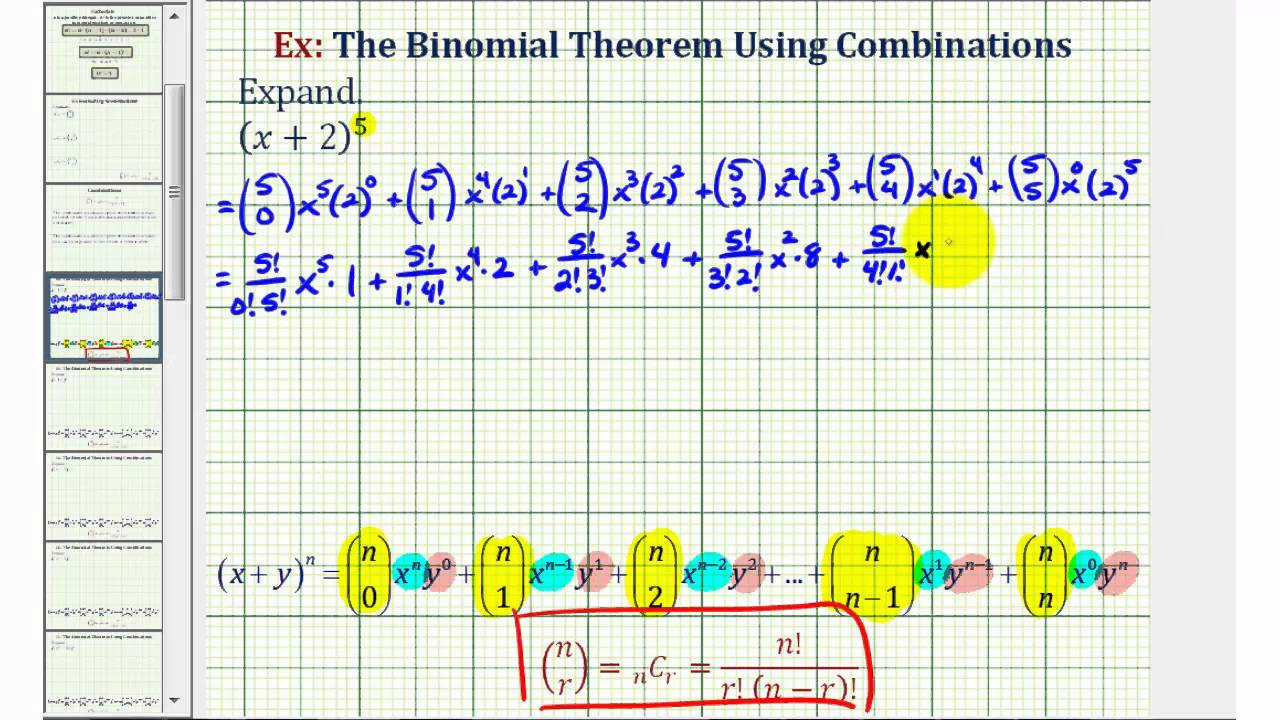
Binomial Theorem Solutions Examples Videos

Unique Quadratic Equation In The Form Y Ax 2 Bx C

Solved Undergraduate Econometrics Problem Set 2 1 Some Chegg Com

Topic 3 Algebra Dr J Frost Ppt Download

3 Expand With The Help Of Formula X Y 3 2

Binomial Expansion Made Easy Expand X Y Algebra Youtube

Expand X 23y 3 Maths Questions

The Expansion Of X Y 2 N Ge 5 Is Done In The Descending Pow

Misc 9 Evaluate X Y X Y Y X Y X X Y X Y Chapter 4 Ncert

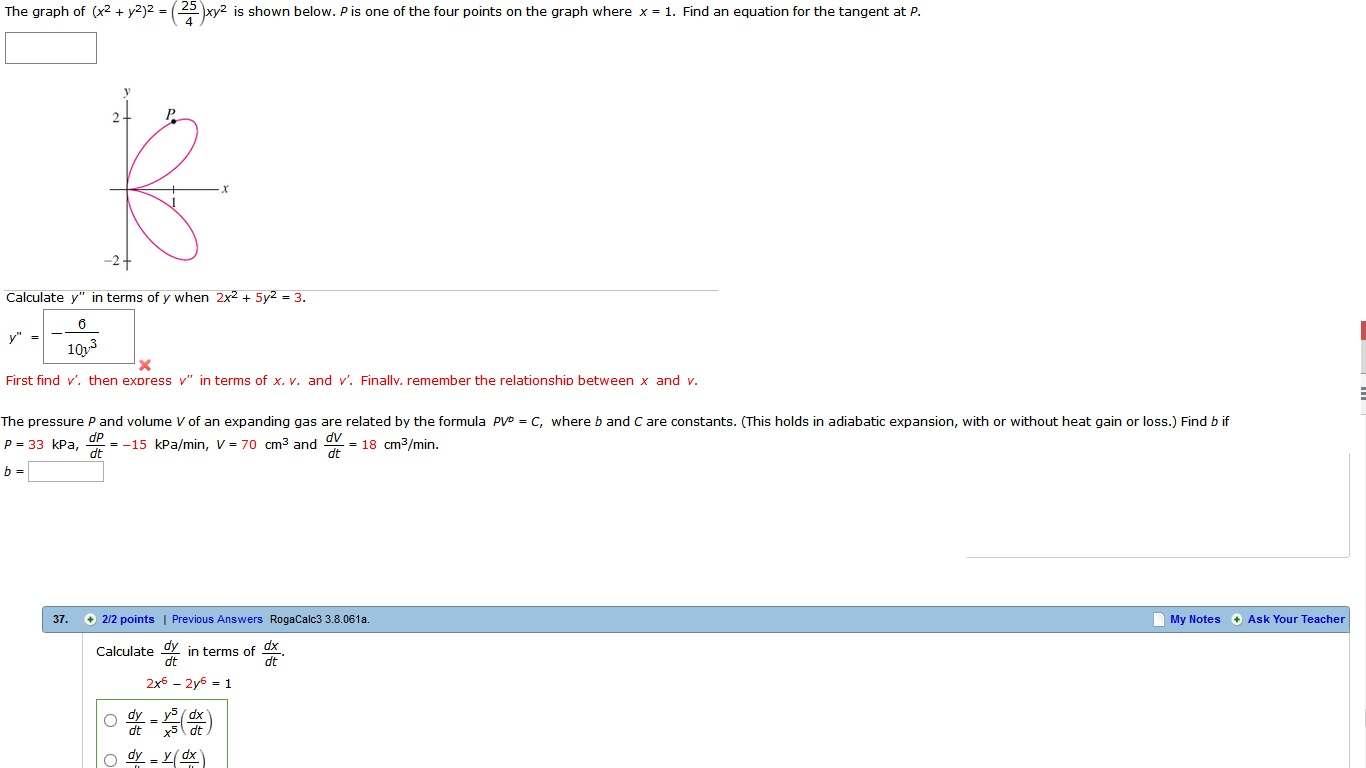
Solved The Graph Of X 2 Y 2 2 25 4 Xy 2 Is Shown B Chegg Com

How Do You Expand X Y 6 Using Pascal S Triangle Socratic

Expand X 1 Y 11 Y 0

How To Expand Using The Identity X Y 3 X3 Y3 3x2y 3xy2 Youtube

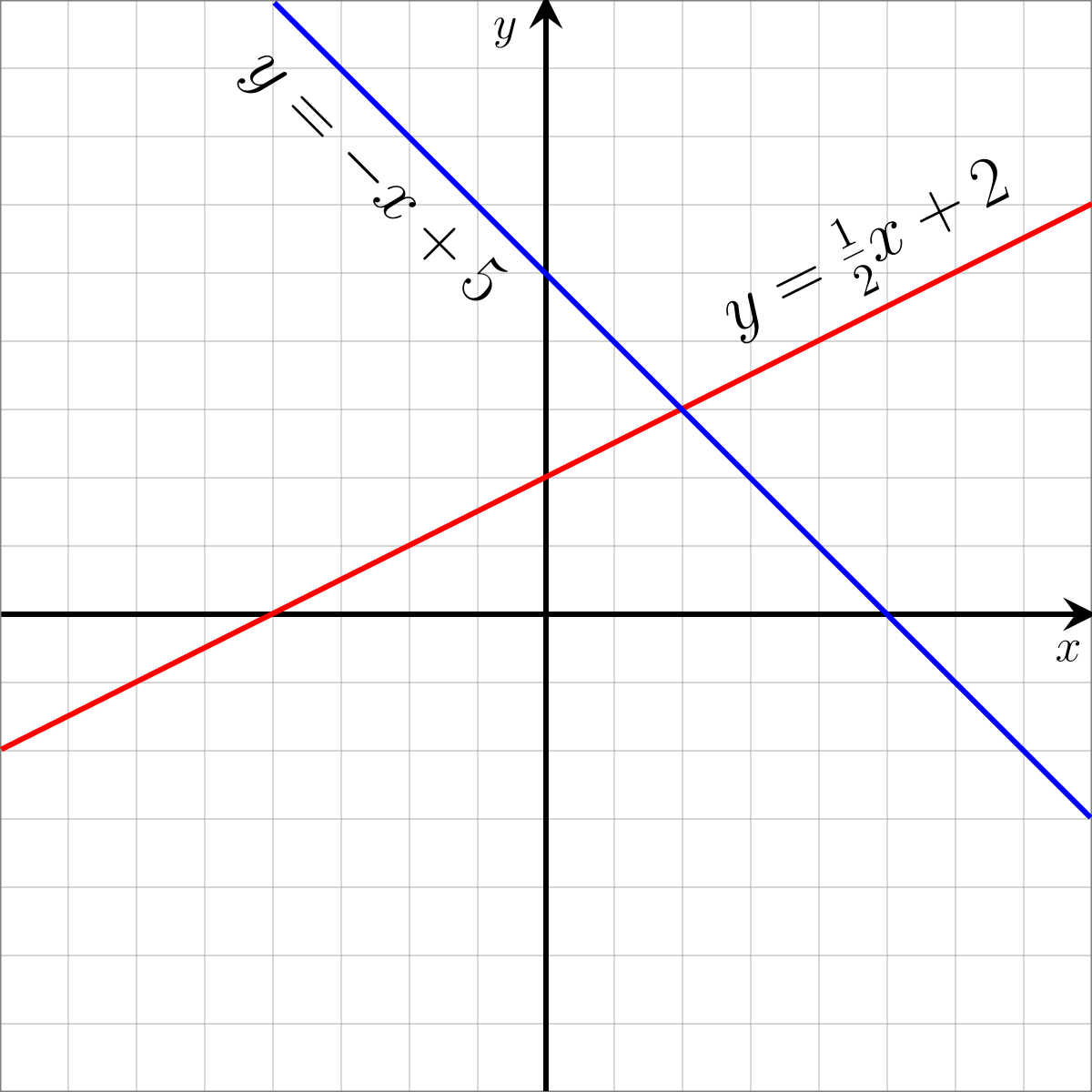
Linear Equation Wikipedia

Binomial Expansion Proof Page 1 Line 17qq Com

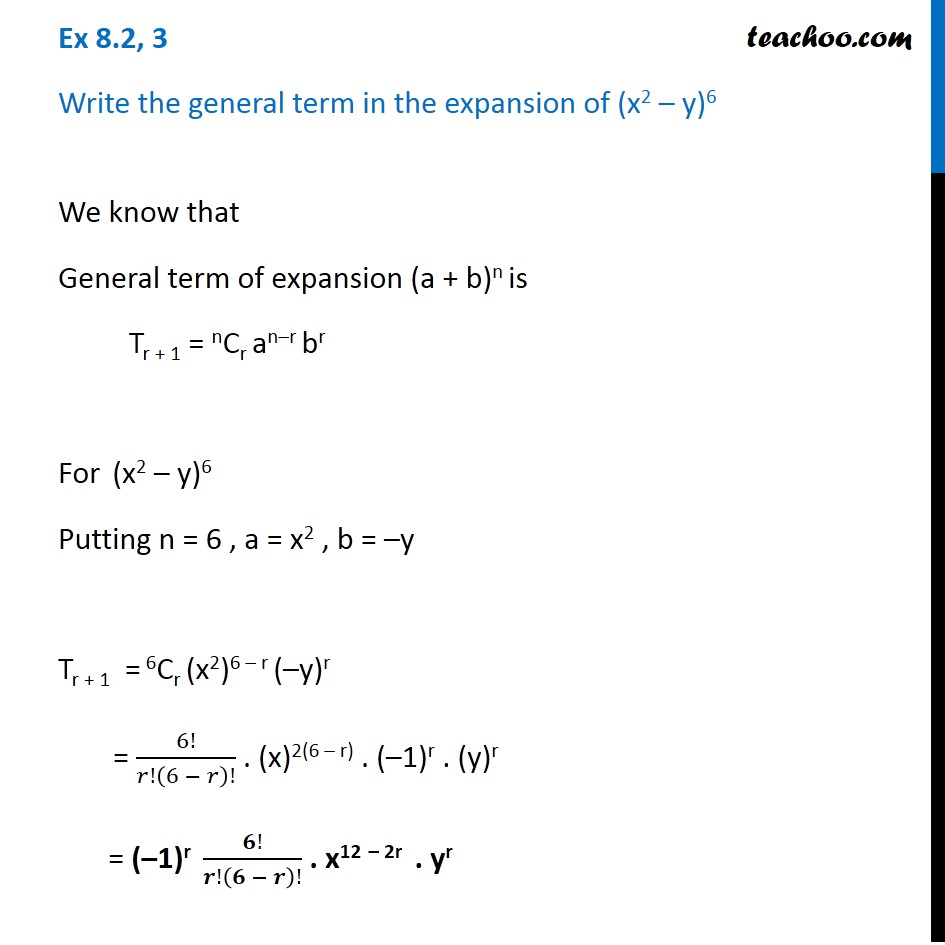
Ex 8 2 3 Write General Term In X2 Y 6 Chapter 8 Ex 8 2

Expand Each Of The Following Using Suitable Identities I X 2y 4z 2 Ii 2x Y Z Youtube

Taylors And Maclaurins Series Mathematical Relations Mathematical Concepts

Expand X Y Z 2 X Y Z 2 Brainly In

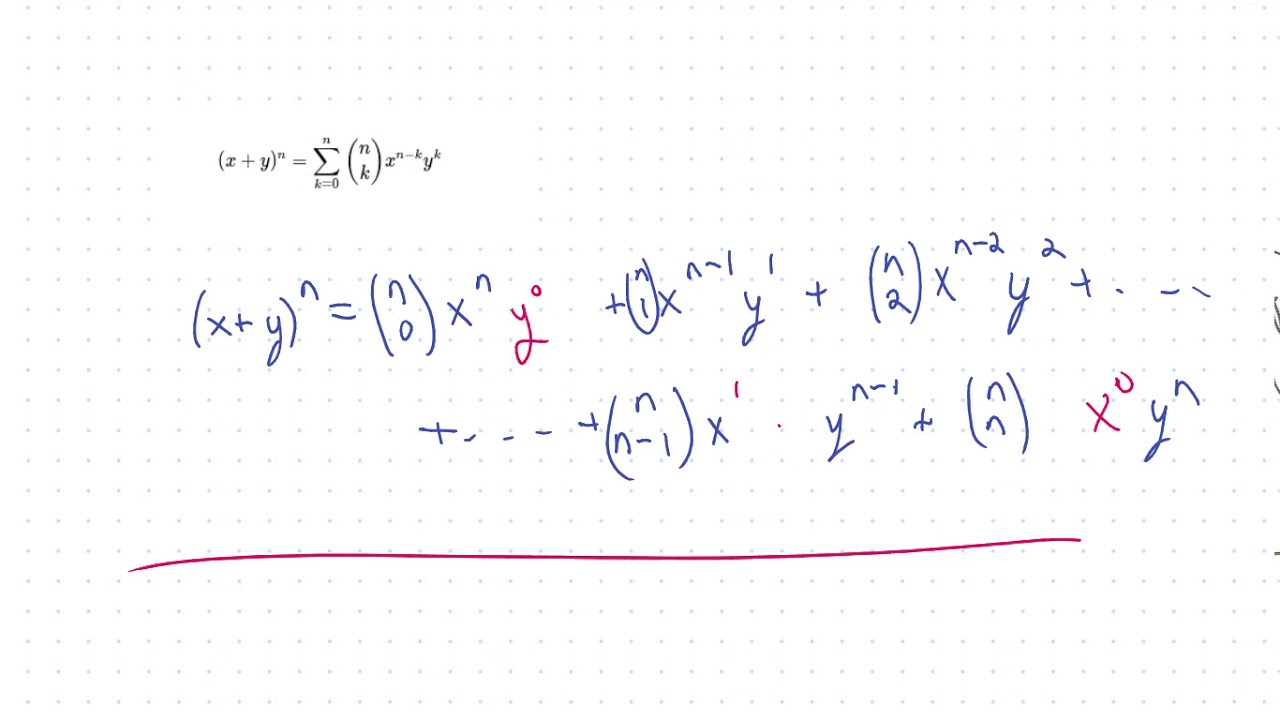
The Binomial Theorem And Combinatorial Proofs

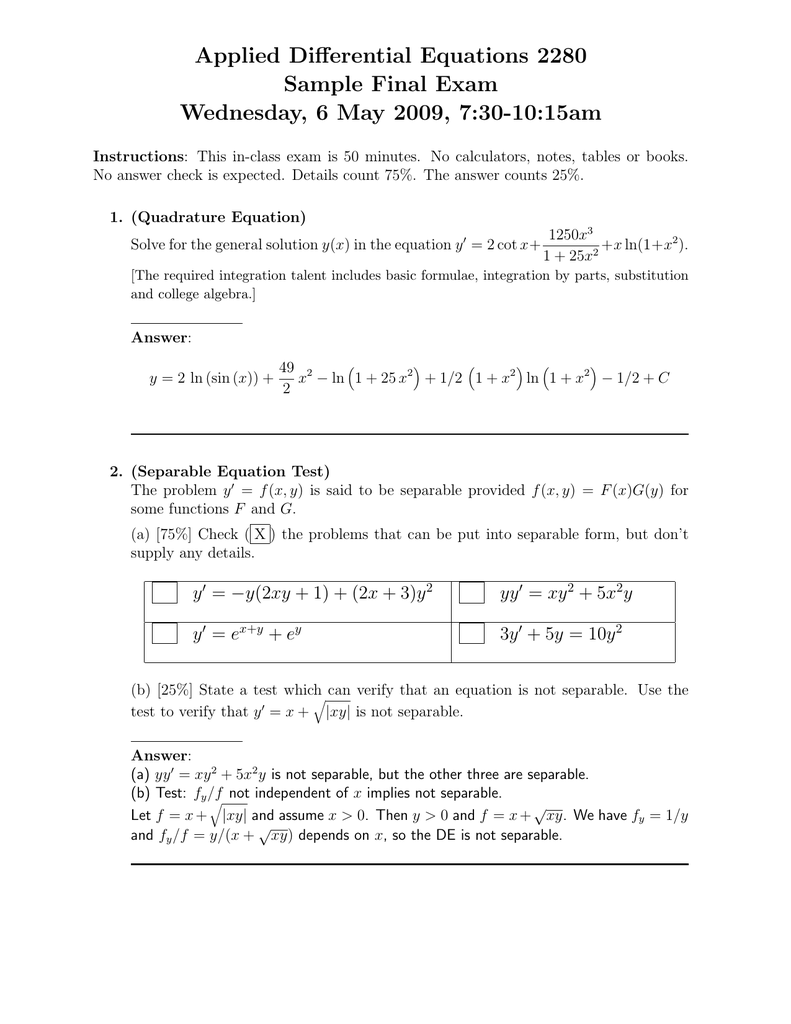
Applied Differential Equations 2280 Sample Final Exam Wednesday 6 May 09 7 30 10 15am

Sat Math Question Are U Supposed To Expand X 4 X 2 And Then Vertex Formula Sat

A Quick And Efficient Way To Expand Binomials Ppt Download

Exponent Rules 7 Key Strategies To Solve Tough Equations Prodigy Education

Solved A I Calculate The Taylor Series Expansion Of The Chegg Com